12/17 Lesson 4.1.5 Putting it all together

1.  Warm-up:  Grab a graphic organizer from your table.

Instructions: Draw lines and/or arrows to show which representations you have learned so far.  Explain the connections between the different representations.  Be sure to include anything you figured out about how the numbers in the equations (rules) relate to tile patterns.

2.  Lesson 4.1.5 Putting it all together (patterns, rules, graphs, tables)

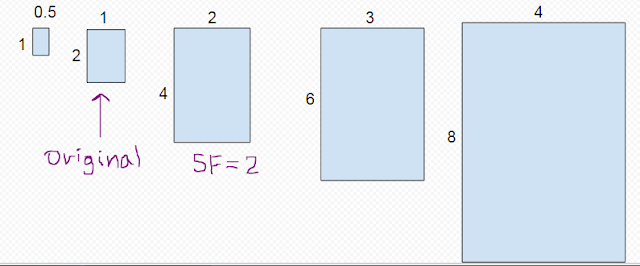
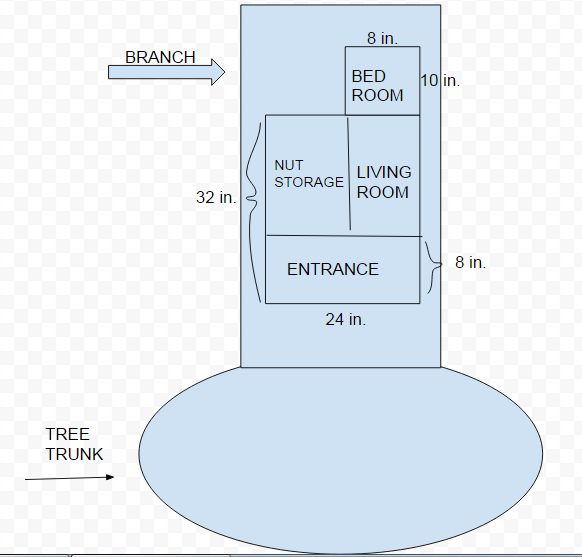
• Challenge Problem #42- For each subproblem:
• Generate the rule
• Create an x to y table
• Create a graph
• Draw the pattern (Figures 0 through 3)
• You may answer the questions in any order, but finish the problem completely before moving on to the next one.

3.  Update your Representations of Patterns Web with any new connections you made after completing this lesson

Homework:  Lesson 4.1.5 8th grade R/P (#44-48 only!)

12/16 Lesson 4.1.4 y = mx + b

1.  Lesson 4.1.4: Introducing y = mx + b

• Do Problems 31 through 33 in teams
• Resource Managers: grab 1 piece of graph paper for each team member
• Do Problems 34 and 35 individually.  After all team members are finished compare your answers.

2.  If you finish the lesson with time remaining, work on the homework for tonight (or tomorrow night)

Homework:  Lesson 4.1.4 R/P

Lesson 4.1.3 and 4.1.4 y = mx + b

1.  Warm-up:  

2.  Lessons 4.1.3 Connecting Linear Rules to Graphs

• Target questions:
• How is growth shown in a graph?
• How is growth shown in a rule?
• How can you determine the number of tiles in Figure 0 from a graph?
• How can you determine which tile pattern grows faster by looking at a graph?
• Problems 22 and 23 in teams (page 159)
• Resource Managers grab books and a resource page for problem 22
• Problem 24 as an individual (silently).  Answer the target questions in your notebook

3.  Lesson 4.1.4: y = mx + b

• Problems 31 through 34 in teams

Homework:  Lesson 4.1.3 R/P 8th grade (answers in GC)

12/14 Lesson 4.1.2 Seeing growth in different representations

1.  No warm-up today

2.  Lesson 4.1.2 Growth in different representations (patterns, equations, graphs)

• For Tile Patterns 1 through 3:
• Give every team member a chance to examine the pattern individually (silently)
• Then, talk about what you see.  How is the figure growing?
• Sketch the next figure (figure 4) and the first figure (figure 0)
• Where are the new tiles being added in the next figure?  Using a colored pencil or highlighter, color in the new tiles in each figure
• Label the figure with a rule (an algebraic equation) where y represents number of tiles and x represents the figure number.
• What will the 100th figure look like?  Describe it in words.  How many tiles will there be?
• Answer the questions in Problem 14.  Be prepared to share your thinking with the class.
• Examine Tile Pattern 4 and answer the questions in Problem 15

Homework:  Lesson 4.1.2 R/P (answers in GC)

12/11 Wrap up Chapter 3 (8th grade)

1.  Warm-up: Distributive Property practice (on side whiteboard)

2.  Snack time! Nom nom

3.  Wrap up Chapter 3

• Checkmark Consensus: Problem 100, 101 on page 135.  Problems 110-112 on page 138

No homework this weekend!

12/10 Lessons 3.2.4 and 3.2.5 Finding patterns, writing rules, and solving equations

1.  Warm-up:  On the piece of graph paper provided on your desk, create an x to y table for the equation y = 2.5x + 1  (see side whiteboard for a hint on how to get started)

2.  How to make a great graph:

• ALWAYS use graph paper
• Use a ruler. Make a neat graph
• Create an x to y table
• Choose an appropriate scale
• Label the x and y axis
• Give the graph a title

3.  Lessons 3.2.4 and 3.2.5 Finding patterns, writing rules, and solving equations

• Problem 99 in teams (on page 135)  Stoplight problem! You may work on problem 100 while waiting for other teams to finish.
• Problem 107 as class discussion
• Problems 108 through 112 in teams (on page 138)

Homework:  3.2.5 R/P (answers in GC)

12/9 Lesson 3.2.3 Using Equations to Solve Problems

New Seats!  We don't need computers today, but log-on anyway to avoid delay in the future

1.  Warm-up: Diamond problems on side whiteboard

2.  Lesson 3.2.2 Using Equations to Solve Problems

• Objectives:
• I will use equations to solve problems
• I will solve equations that can't be solved with algebra tiles (rational coefficients)
• Problems 89-92 in teams (page 131) using Check mark Consensus
• Stoplight! You may work on your R/P while waiting for other teams to finish
• Problem 93 guided by Mr. Robinett
• How to make a great graph:
• ALWAYS use graph paper
• Use a ruler. Make a neat graph
• Create an x to y table
• Choose an appropriate scale
• Label the x and y axis
• Give the graph a title

Homework:  Lesson 3.2.3 R/P (answers in GC)

12/8 Lessons 3.1.1 and 3.1.3 Finding Patterns and Writing Rules

1.  Warm-up: 

2.  Lessons 3.1.1 and 3.1.3 Finding Patterns and Writing Rules

• Objectives:  
• I will investigate patterns to determine a rule
• I will express the patterns that I find in an x to y table, an algebraic expression, and a graph
• Resource page problems 3-2 and 3-3
• In teams, determine the pattern, fill in the missing values, and write a rule (in words or algebraically)
• Check the answer key in Google Classroom
• Resource page problem 3-18 (on the back)
• Draw Figure 0 and Figure 4, fill in the x to y table, find algebraic rule, graph the data
• Check the answer key in Google Classroom

Homework: 3.1.1 R/P (8th grade)

12/7 Lesson 3.2.2 Guess My Number

1.  Warm-up:  

I'm thinking of a number that...

• When you double my number
• then add 8
• then subtract my number
• and subtract 4

You get...

• My number plus 4

Guess my number.  Check that your solution is correct.  Then, write an algebraic equation to represent the situation.

2.  Lesson 3.2.2 Guess My Number

• Objectives:
• I will represent word problems with algebraic equations
• I will determine how many solutions an equation has
• Guess my Number game
• Problem 81 in teams:  How many solutions does each equation have?  Check your solution if applicable.
• Individually: Make your own Guess my Number Game.  You must know the solution to your own game!
• Share your game with your teammates to solve.

Homework:  Lesson 3.2.2 R/P (8th grade) #86-88 only

12/4 Lesson 3.2.2 (8th grade) Guess My Number

1.  Return Unit 4 Tests

• Feedback
• Re-collect

2.  Math Attitudes Survey (click the link)

3.  Lesson 3.2.2 Guess My Number

• Objectives:
• I will represent word problems with algebraic equations
• I will determine how many solutions an equation has
• Guess my Number game
• Problem 81 in teams:  How many solutions does each equation have?  Check your solution if applicable.

Homework:  Lesson 3.2.2 R/P (8th grade) #86-88 only

12/3 Unit 4 Test

After you have finished the test, turn it face down on your desk and:

• Try a logic puzzle from this website (click the link)
• Or a Calcudoku puzzle, also known as Ken-Ken (click the link)
• Read silently

12/2 Team Test & Study Guide

1.  No warm up. We have too much to do, and too little time! You will need to log on to a computer

2.  Questions about Closure problems?  These types of problems will be on the test, so now is the time to ask.

• Correct Closure problems tonight (if you haven't already).  Use the table at the end of the Closure section to guide your studying tonight.

3.  Finish Team Test

• Add your equation and a picture of your finished GO to our class document in Google Classroom.
• Try to solve other teams' equations.  Use their GO if you get stuck.
• This document is a great study tool for tonight

Homework:  Study for the Unit 4 test tomorrow

12/1 Unit 4 Team Test

1.  Warm-up:  Checking solutions (see side whiteboard)

2. Announcements:

• Mistakes I'm noticing
• Math Counts meeting today at lunch
• Unit 3 Project feedback

3.  Team Test: Be the Teacher!

• From the 8th grade book Ch. 2 Closure on page 84
• Design one problem that would allow you to assess students' understanding of the major concepts from Ch. 2

4.  Unit 4 Closure problems:

• 8th grade book Ch. 2 Closure #91, 93, 95
• 7th grade book Ch. 6 Closure #145

Homework:  Finish Unit 4 Closure problems (see above)

11/30 Finish Lesson 2.1.9

1.  Entrance Ticket- Solve:  4 - (2x + 6) + 3x - 1 =  2x + 5

• Record your process
• Check that your solution is correct

2.  Announcements:

• Unit 4 Test this Thursday (12/3)
• Math Counts:  Interested on being on the LOMS Math team? Meet with Mr. Kirby @ lunch tomorrow for more details

3.  Lesson 2.1.9 Solving equations

• Finish Problem 83 (page 85)
• Do Problem 89 in class (part of the R/P)
• Additional Challenge: 9th grade Algebra problem on the white board

Homework:  Lesson 2.1.9 R/P

11/20 Lessons 2.1.8 and 2.1.9 Solving Equations

1.  Warm-up:  Stick puzzle on side whiteboard

2.  Lessons 2.1.8 and 2.1.9

• Objective:  I will use "legal moves" to simplify and solve algebraic equations
• Problem 75 (page 78) and Problem 83 (page 81) in teams.
• Always check that your answer is correct by substituting your solution for "x" back into the original equation
• Hint: Some equations might have no solution.  What do these types of equations have in common?

No Homework over the break! Have a safe and relaxing holiday!

11/19 Lesson 6.1.4 Solving, writing and graphing inequalities

1.  Warm-up:  Yesterday, we came across some scenarios in which the 3 "legal moves" couldn't be applied, yet the expressions weren't fully simplified.

Two such scenarios are posted on the side whiteboard.  For each scenario, create a legal move that would simplify the expressions into a single variable on one side, and a collection of unit squares on the other.

2.  Lesson 6.1.4 Solving, writing and graphing inequalities.

• Objective:  I will solve, write and graph inequalities related to real-world problems
• Team Check marks!  Problems 36 through 40

Homework:  Lesson 6.1.4 (7th grade) R/P

11/18 Lesson 6.1.3 Solving and Graphing Inequalities

1.  Warm-up:

a)  Which expression is larger, Mat A or Mat B?

b)  Find at least 4 values of x that would make Mat A larger.

2.  Lesson 6.1.3 Solving and Graphing Inequalities

• Objective:  I will solve and graph inequalities using Expression Comparison Mats
• Problem 26 as class discussion + Mini-lesson
• Build-Simplify-Graph cooperative learning worksheet

Homework:  Lesson 6.1.3 R/P

11/17 Unit 3 Project Gallery Walk

1.  Warm-up:  On Problem #1 of yesterday's Sage and Scribe activity, we were left with the following expressions:

Left expression                      Right expression
        x                              1

Which is greater?  What additional information do you need to know?

What if the Left expression is 5y and the Right expression is 4y?


2.  Unit 3 Project Gallery Walk

• Half the class sets up, the other half walks around the room
• Respect people's projects.  Do not touch until given permission.  
• Ask questions.  If you are displaying your project, be ready with answers
• Leave constructive feedback
• A positive comment followed by a suggestion

3.  Unit 3 Project Turn-in

• Paper turn-in vs. Digital turn-in

4.  Finish Sage and Scribe activity

Homework:  Lesson 2.1.7 R/P (answers in GC)

11/16 Lesson 2.1.7 (8th grade) Simplifying and Recording Work

1.  Warm-up:  Use Algebra Tiles to compare the following expressions

Which is greater:   −1 + 6y − 2 + 4x − 2y  or  x + 5y − (−2 + y) + 3x − 6

2.  Lesson 2.1.7 (8th grade) Simplifying and Recording Work

• Objective:  I will simplify expressions and record my process
• Cooperative learning activity: Sage and Scribe
• Sage builds original expressions, then simplifies using "legal moves"
• Scribe records simplified expressions, one step at a time
• Switch after completing a problem

3.  Closure:  Problem #1-  Which side is greater?  What information would you need in order to answer this question?  For which values of x would the left side be greater?  The right side?

Homework:  Finish Unit 3 project! 

11/13 Lesson 2.1.5-6 Which is Greater?

1.  Warm-up:  Simplify the following expressions using the Expression Mat.  Record each step using the strategy we practiced yesterday (see below).

a)  4x -(x² + y -4) + x² -3            b)  2y + 4x - 3y - (4 + 2x) - x² + y² + 3

• Strategy:
• Build original expression.  Record original expression
• Move all tiles to "+" region.  Record new expression
• Remove zero pairs. Record simplified expression

2.  Announcements:

• Weekly Privilege Prize
• Unit 3 Project due Tuesday

3.  Homework problem from last night:  3d² - 1, for d = -5

4.  Lesson 2.1.5 and 2.1.6  Which is Greater? (starts on page 65)

• Objective:  I will determine which expression is greater using "legal moves" to simplify expressions.
• Problem 47 as a mini-lesson:  Which is greater?
• Problem 48 in teams. Stoplight!  Do not move ahead
• Discussion:  What are "legal moves?"
• Problems 56 and 57 in teams

5.  Closure:  Problem 57, part b)   Can you tell which expression is greater? Why or why not?

Homework:  Work on your Unit 3 project (details in GC)

11/12 Lesson 2.1.4 Simplifying Algebraic Expressions

1.  Warm-up:  Using only unit tiles, make "zero" on your expression mat.  How many ways can you accomplish this task?

2.  Lesson 2.1.4 Simplifying Algebraic Expressions

• Objective:  I will simplify algebraic expressions by removing zero pairs
• Problem 36 as mini-lesson
• Strategy:
• Build original expression.  Record original expression
• Move all tiles to "+" region.  Record new expression
• Remove zero pairs. Record simplified expression
• Problems 37 through 39 in teams

3.  Closure:  Describe the different ways you can represent zero using your Expression Mat.  Include an example and draw a picture.

Homework:  Lesson 2.1.4 (8th grade) R/P

11/10 Introduction to the Expression Mat

1.  Warm-up:  What does  " - " mean?  Find as many ways as you can to describe this symbol and discuss how these descriptions differ from each other.

Announcements:

• Unit 3 Project (details in GC) due next Tuesday

2.  Lesson 2.1.3 (8th grade)  Intro to Expression Mats

• Objective:  I will represent expressions in multiple ways
• Expression Mat Mini-lesson
• Do Problems 25 through 27 in teams (check mark on side whiteboard)

Additional Challenge Problems (on front white board)

Homework:  Lesson 2.1.3 R/P (due Thursday)

11/9 Lesson 2.1.2 Simplifying Algebraic Expressions

1.  Warm-up:

For the following problems:

• Build the expression with Algebra Tiles
• Simplify the expression by grouping like terms (if possible)
• Write the name of the simplified collection of tiles

2.  Lesson 2.1.2 Simplifying Algebraic Expressions

• Objectives:
• I will find the perimeter of Algebra Tile models
• I will simplify algebraic expressions by combining like terms
• Problems 13 and 14 in teams
• Additional Challenge: Problem 15

Homework:  Lesson 2.1.2 R/P (answers in GC)

11/5 Lesson 2.1.1 (8th grade)

1.  Warm-up:  Review Team Test with your team.  Do not log-on to computers.  We will change seats in a moment

2.  Weekly Privilege Prize:  No weekend homework!

3.  New Teams, new seats, new computers

4.  Lesson 2.1.1 Intro to Algebra Tiles

• Objectives:
• I will learn the names of the different types of tiles
• I will combine like terms using Algebra Tiles
• Introduction to Algebra Tiles

5.  Closure:  Explain when tiles can and cannot be combined

Homework:  Lesson 2.1.1 R/P (8th grade)

11/3 Finish Lesson 5.2.6

1.  Warm-up:  Is it a fair game?

2.  Evens and Odds discussion

• After looking at the mystery spinners (click the link),  how did it change our initial predictions?
• What is the actual theoretical probability of spinning even?  Odd?
• Showcase of multiple methods

3.  Unit 3 Project announcement

4.  Finish Lesson 5.2.6 (Problem 82) 

Homework:  Lesson 5.2.6 R/P #88-92

                     Unit 3 Project (due 11/17)

11/2 Lesson 5.2.6 Probability of Compound Events

1.  No Warm-up today.  Begin working on Lesson 5.2.6 in teams

2.  Lesson 5.2.6 Probability of Compound Events

• Do Problems 77 and 78 in teams (16 minute timer)
• Finished early?  Work on R/P
• Game Time:  Odds and Evens
• Work with your team to build a probability table.  Which result is more likely, odd or even?

Homework:  Lesson 5.2.6 core problem # 78 (if not finished in class), and R/P #83-87

10/30 Lesson 5.2.5 Probability Trees

1.  Warm-up:  Using the Probability Tree from the Crazy Creations Ice Cream store, answer the following questions:

a)  What is P(chocolate and sprinkles)?

b)  What is P(vanilla and grape)?

c)  What is P(hazelnuts, chocolate, and plum)?

d)  What is P(toffee bits and sprinkles)?


2.  Announcements:

• We will finish Unit 3 on Tuesday
• Team Test on Wednesday

3.  Finish Lesson 5.2.5 Probability Trees

• Notes/discussion:  Mutually exclusive events
• Do Problems 68 and 69 in teams

4.  Probability Challenge problem (extra credit, due Monday)

Design two number cubes that meet the following requirements:

• P(sum is 9) = 1/6
• P(sum is odd) = 2/3
• P(sum is divisible by 5) = 1/18

Hints/Tips:

1. The number cubes don't need to be exactly the same
2. The number cubes don't need to go up in ascending order
3. A number can be repeated on a number cube
4. All faces of the number cube must contain positive integers

10/29 Lesson 5.2.5 Probability Trees

1.  Warm-up:  Design a Ten O's board with your team

2.  Lesson 5.2.5 Probability Trees

• Objective:  I will develop visual models for counting outcomes of multiple events
• Do Problem 65 in teams
• Finished early?  Change the problem so that instead of finding the sum, you find the product of the 2 spins.  Which result is most likely now?
• Problem 66 a) individually
• Complete Problems 66, 67 using Probability Tree resource page
• Use either a Probability Tree or Table to solve Problems 68 and 69

Homework:  Lesson 5.2.5 R/P

10/28 Lesson 5.2.4 Probability Tables

1.  Warm-up:

Ask yourself this question: "Does the previous result affect what will happen next?"


2.  Lesson 5.2.4 Probability Tables

• Objective:  I will develop a method for counting and organizing all possible outcomes
• Ten O's game
• Analysis of Ten O's game using a probability table (Problems 55 through 57)
• Place a check-mark on the board when your team has completed a problem
• Create your own probability table to solve Problems 58 and 59

Homework:  Lesson 5.2.4 R/P

10/27 Lesson 5.2.2 and 5.2.3

1.  Warm-up:  Read problem 36 below and answer the questions that follow.

Janelle’s aunt and uncle have three children, two of whom are girls.  Assuming that girl children and boy children are equally likely, Janelle thought that the chance of having two or more girls out of 3 children must be 50%.  Janelle’s brother thought the chance of having so many girls had to be less than 50%.  

a)  Make a conjecture.  What do you think the probability of having two or three girls in a family of three is?

b)  Design a computer simulation that would help you solve this problem.

2.  Finish Lesson 5.2.2 (page 260)

• Complete Problems 36 and 37 in teams
• 20 minute timer--if you don't finish, it's homework

3.  Start Lesson 5.2.3 Compound Independent Events (page 263)

• Objectives
• I will determine whether an event is dependent or independent
• I will calculate the probability of compound independent events
• Problem 43 in teams. Stoplight.  Check with Mr. Robinett before moving on
• Problem 44 and 45 in teams.  Stoplight. Check with Mr. Robinett before moving on

Closure:  Summarize your understanding of independent and dependent events.  How can you determine if two events are independent of one another?

Homework:  Lesson 5.2.3 R/P (skip 51, 53) and Lesson 5.2.2 Problems 36 and 37

10/26 Lesson 5.2.2 Probability using Computer Simulations

1.  Warm-up:

For each of the following games, a random number generator is used.  The random numbers are integers ranging from 1 to 20.  Assume that Player Y wins if Player X does not.

Game 1:  Player X wins if the number is prime
Game 2:  Player X wins if the number is even
Game 3:  Player X wins if the number is NOT divisible by 3

For each game:

a) Find the Probability that X wins        b) Find the probability that Y wins     

c) Is it a fair game?


2.  Lesson 5.2.2 Probability Using Computer Simulations

• Objective:  I will calculate the probability of complex situations using computer simulations
• Mr. Robinett leads Problem 35.  When your team is finished, fill out this form to enter your team's data.  Only 1 form per team!
• With your new knowledge of computer simulations, design an experiment to help you solve Problems 36 and 37

Homework: Lesson 5.2.2 R/P

10/23 Lesson 5.2.1 Is It a Fair Game?

1.  Warm-up: Stick math (on the side whiteboard)

2.  Lesson 5.2.1 Is it a fair game?

• Objectives:
• I will calculate simple probability and complementary probability
• I will determine if a game is fair using my knowledge of probability
• Do Problems 23 through 27 in teams
• Challenge:  Modify the game in Problem 25 to make it a fair game

10/22 Lesson 5.1.2 What is the Percentage of the Whole?

1.  Warm-up:  Quick write.  How did it go yesterday?  Do you understand how to calculate percent using a diagram?  Rate your understanding on a scale from 1 to 5, where 1 is "I don't get it at all. Help!" and 5 is "I totally understand. I could teach this topic to others."

Whole-class debrief

2.  Lesson 5.1.2 What is the Percentage of the Whole?

• Objective:  I will calculate discount (sale) prices using my knowledge of percentages
• Discussion:  Think about your experience shopping at a sale.  How have you seen prices advertised?  How do you determine if you are getting a great deal?
• Do: Problems 14-17 in teams.
• Put a check under your team number after everyone in your team completes a problem (and agrees on an answer)

3.  Closure:  How might you find the sale price of an item that was discounted 13% without using a visual model?

Homework:  Lesson 5.1.2 R/P (answers in GC)

10/21 Lesson 5.1.1 Finding Percentages

Lesson 5.1.1 Finding Percentages

• Objective:  I will use visual models to solve a variety of percent problems.
• In your teams, complete problems 1 and 4-8.

Homework:  Lesson 5.1.1 R/P

10/20 Lessons 4.2.3 and 4.2.4 Unit Rate, Writing Equations

1.  Warm-up:

a)  In order for a graph to represent a proportional relationship, there are 2 requirements.  Name them.

b)  Addressing misconceptions:  Yuna is 4 years older than her sister, Debbie.  The relationship between Yuna's age and Debbie's age is not proportional.  Prove it using either a table or a graph.

c)  Check out your quiz score in PowerSchool


2.  Lessons 4.2.3 (page 207) and 4.2.4  Calculating Unit Rates and Writing Equations

• Objectives:  
• I will calculate the unit rate of various proportional relationships
• I will write equations to represent proportional relationships
• Mini-lesson:
• Unit rate/ unit price
• Proportional relationship general equation:  y = k · x
• Do problems 46, 48, 49 (Lesson 4.2.3) and 55 through 57 (Lesson 4.2.4) in teams.  Any problems you do not finish are homework.

Homework:  See above

10/19 Lesson 4.2.2 Proportional Relationships in Tables and Graphs

1.  Warm-up:  Remember Kaci the cheese lover?  Well, here's a graph (thanks, Gabriel) to represent the cost of her 2 favorite cheeses.

Here is a link to the tables and graph.

Black = Mozzarella       Gold = Havarti

  a)  Compare the two graphs.  What is the same, and what is different?

b)  Which cheese is more expensive?  How can you tell by looking at the graph?  How can you tell by looking at the table?

c)  What is significant about the point (1, y) on each of the graphs?









2.  Lesson 4.2.2

• Objective:  I will be able to determine whether or not a relationship is proportional by examining a table or graph.
• Complete problems 36 through 39 with your team.

3.  Proportional relationships quiz

• Socrative.com --> Login as a student --> Room code: Robinett

Homework:  Lesson 4.2.2 R/P (answers in GC)

10/16 Lesson 4.2.2 Proportions in Tables and Graphs

1.  Warm-up:  Compute the area and perimeter of each rectangle.  Also, describe the rectangle in terms of its scale factor (see example).

2.  How do enlargements and reductions affect perimeter and area?  Can we describe the change using a general rule or equation?

• Hypothesis wrecking

3.  Lesson 4.2.2 Proportions in Tables and Graphs

• Objective:  I will be able to determine whether or not a relationship is proportional by examining a table or graph.
• Mini-lesson:  How to access and use Desmos Graphing Calculator
• Click this link to access the tutorial table/graph.
• Problem 35: each team member should use the eTool to complete the table and graph
• Answer questions d) through f) in your notebook.  Part f) is very important.
• Do Problems 36 through 39 with your team

Homework: Lesson 4.2.2 R/P (due Tuesday)

10/15 Lesson 4.2.1 Proportional Relationships

1.  Warm-up:

2.  Lesson 4.2.1 Proportional Relationships

• Objective:  I will examine whether or not a relationship is proportional
• Team Discussion (no writing):  Problems 22 and 23
• Class Discussion using eTool: Problem 24
• Use eTool (no writing) to complete Problem 25
• Team Discussion (write answers): Problem 26

3.  Pattern Finding: What happens to the area of a shape when it is enlarged or reduced?

• Hypothesis Wrecking

Homework:  Lesson 4.2.1 R/P (answers in GC)

Team Quiz + Finish 4.1.2 and 4.1.1

1.  Warm-up:

• Read the scale drawings quiz prompt (below)
• Decide on a scale with your team.  You must all use the same scale

2.  Scale Drawings Quiz

Professor Nut is designing a tree house.  The branch that he is building on has a width of 28 inches and a length of 70 inches.  For safety reasons, the walls of his tree house should end 2 inches prior to the edge of the branch.  He wants the entrance of the tree house set 10 inches from the trunk.  He wants his Winter Nut Storage room to have twice the area of his living room.

Professor Nut's nutty, not-to-scale drawing:

3.  Finish Lessons 4.1.1 and 4.1.2

• 4.1.2 Problem #15 on page 193
• 4.1.1 Problem #5 on page 189
• Extension:  Also make enlargements/reductions using the scale factors of 2, 4, and 1/2.  How do the perimeters and areas compare to the original drawing?  Do you see a pattern?
• Check your answers in the Top Secret Answer Key on the computer cart

No homework tonight!

10/13 Lesson 4.1.2 Scale Drawings

1.  Warm-up:  Of the 4 vocabulary words we learned yesterday, (reduction, enlargement, scale factor, similar figures) choose 1 or 2 that you feel comfortable explaining.  Prepare a short explanation to share with your classmates.

2.  Lesson 4.1.2 Scale Drawings

• Lesson Objective:  
• I will create scale drawings using the scale factor
• I will compute actual lengths and areas from scale drawings
• Maps and blueprints are examples of scale drawings

Do: Problems 12 through 15 (page 192)

Finished early:  Go back and finish Lesson 4.1.1

Homework:  Lesson 4.1.2 R/P (answers in GC)

10/12 Lesson 4.1.1 Scale Factor

1.  Warm-up:  In the classwork section of your notebook, copy down today's key vocabulary.  Write what you think the definition of each might be.

2.  Lesson 4.1.1

• Key Vocabulary:
• Enlargement
• Reduction
• Scale Factor
• Similar Figures
• Objective:  I will be able to enlarge and reduce drawings given the scale factor.
• Do: Problems 1 through 5

3.  Closure:  Write 2 sentences that use today's vocabulary words in context.  Use all 4 vocabulary words.

Announcements:  

• Minecraft application form is due by the end of school today.  See blog post from Friday

Homework:  Lesson 4.1.1 R/P (answers in GC)

10/9 Return Tests + Puzzle Investigator

1.  Warm-up:  Stick-Math Puzzle on side whiteboard

2.  Minecraft Tournament:

• Application form

3.  Return Individual Tests and Team Tests

4.  10 minutes to:

• Apply for Minecraft tournament selection
• Ensure ILP is complete i.e. all screenshots are uploaded
• Review both tests with teammates and friends

5.  Sierpinski Triangle debrief

• Share your fractal creations with your team
• Talk about the patterns you found with regards to the shaded area
• How can you use that pattern to determine what (3/4)⁰ is?
• Don't worry if you can't yet... we'll get there eventually as we move into 8th grade math.

10/8 Individual Test

When you are finished with your test, you may:

• Work on Chapter 3 ILP 
• does your Puzzle Investigator look like this?
• Play one of these math games.

Chapter 3 ILP is due tomorrow.

10/7 Unit 2 Closure and Study Guide

1.  Warm-up:  Rewrite each percent as a fraction and decimal:

• 12%
• 132%
• 0.5%

Also, do Closure 3-137 in Chapter 3.

2.  Grade Closure problems

• Put a star for mastery, an "x" for needs improvement

3.  Make Individual Study Guide

• Find your study guide in Google Classroom
• Use the table at the end of the Ch. 2 & 3 Closure section to help you form your study plan
• Turn in your study guide after you have finished creating it.

4.  Mini-groups & Individual study time

Reminder: Individual Test tomorrow, Ch 3 ILP due Friday

10/6 Unit 2 Team Test

1.  Unit 2 Team Test

• Mr. Robinett will grade 1 teammate's paper and the entire team will receive that grade.  Work together.  No one behind, no one ahead.
• Justify your reasoning when necessary.  For example, how do you know you have created the smallest possible number?

2.  Work on Closure problems (due tomorrow) or Chapter 3 ILP (due Friday)

Homework:  Chapter 2 Closure #132, Chapter 3 Closure # 131, 132, 134, 136 due tomorrow

10/5 Simplifying Expressions

1.  Warm-up: Solve the following using mental math (no need to write anything down)

• 3 · ¹/₃ · 4 · 7 · -¹/₅ · 0 · 6 · 3
• 4,556 - 10 - 4,500
• ¹/₆ · (-4) · 3 · 6

2.  Today, we will practice efficiently simplifying expressions.  Efficiently is the key word!  Many times, we can use mental math to break the problem down into small, easy chunks.

Go to Google Classroom and find the document "Efficiently Simplifying Expressions"

You may work independently or with a partner.

3.  Simplifying Expressions quiz

Go to Socrative.com, click Student login, and type in my room code: Robinett

This is a timed quiz. Remember the key word? Efficiency is the key to finishing with time to spare.

Homework:  Chapter 2 Closure #132, Chapter 3 Closure # 131, 132, 134, 136 due Weds.

10/2 Lesson 3.3.3 Simplifying Expressions

1.  Warm-up:  Lesson 3.3.3, problem 3-114 (projected on the screen)

• Early finishers:  Try the Challenge problem 3-117

2.  Lesson 3.3.3 Simplifying Expressions

• Objective:  I will use the Commutative and Associative Properties to simplify expressions.
• Mr. Robinett leads problem 115
• Now you try:
• -7 - 8 - 4 + 12 - 8 + 16
• -6 - 3 · (-1) + 3 · (-4)
• 3 - 4 · (-7) - 3 · (-4) - 25
• ¹/₂ - 3 · (-¹/₃) - ¹/₈ · 4
• In groups:  Problems 116 through 118

Homework:  No Review/Preview! Instead, do Ch. 2 Closure #132 and Ch 3 Closure #131, 132, 134, 136

10/1 Lesson 3.3.2 Dividing Decimals

1.  Warm-up:  Solve the following problems using the Super Giant One:

• 12 divided by ³/₄
• 3 ¹/₈ divided by 1 ¹/₄

2.  Lesson 3.3.2 Dividing Decimals

• Objective:  I will develop strategies for dividing decimals (without using a calculator).
• Whole class:  Problem 3-98 and 99
• Groups Do: Problem 100-105

Homework: Lesson 3.3.2 R/P

9/30 Lesson 3.3.1 Division of Rational Numbers

1.  No warm-up today.  Please grab a textbook and work with your team to complete Lesson 3.1.1

Mr. Robinett expects you to work hard today, despite his absence.  The top-performing groups will be awarded Dojo points!


2.  Lesson 3.3.1 Division of Rational Numbers

• Objective: 
• I will practice dividing rational numbers (fractions, decimals, and mixed numbers).  
• I will investigate multiple methods for dividing rational numbers
• Do: Problems 3-87 through 92

3.  Closure:  Which method (Visual model, common denominator, Super Giant One) works best for you?  Does it depend on the problem?

Homework:  Lesson 3.3.1 R/P

9/29 Lesson 3.2.5 Operations with Integers

1.  Warm-up: The Game!

-3 + 4 · 2 + -8 · -3 + -1

• Use grouping symbols to create an expression with the highest total
• Use grouping symbols to create an expression with the lowest total

2.  Lesson 3.2.5 Operations with Integers

• Tug-O-War eGame
• Record your "moves" a.k.a expressions in your notebook
• Essential Question:  What is your strategy?
• Discuss your strategy with your team.  Then, we will have a whole class discussion
• Do: Problems 80 and 81

Homework: Lesson 3.2.5 R/P

9/28 Chapter 3 ILP

1.  Sign in to Gmail and find your Chapter 3 ILP

2.  Introduction to Chapter 3 ILP

• AM = "Almost Mastered"
• The Rocked It activity is optional.  Do it if you think you need the extra practice
• Puzzle Investigator- "Sierpinski Triangle" is mandatory for everyone.
• Please follow the PI protocol (on page 5 of your ILP)
• Take a screenshot of your work and paste it in your ILP
• Due Friday, October 9th

3.  Begin work on Chapter 3 ILP

No Homework tonight!